
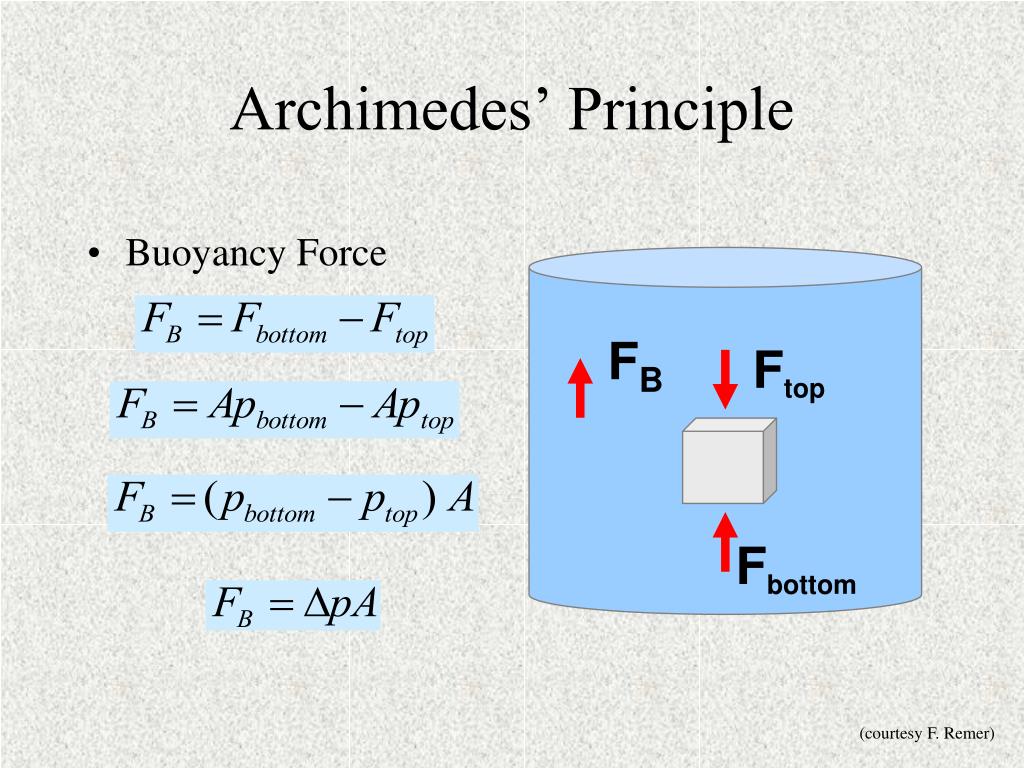

The volume submerged equals the volume of fluid displaced, which we call V fl. The fraction submerged is the ratio of the volume submerged to the volume of the object, or We can derive a quantitative expression for the fraction submerged by considering density. In Figure 4, for example, the unloaded ship has a lower density and less of it is submerged compared with the same ship loaded. The extent to which a floating object is submerged depends on how the object’s density is related to that of the fluid. Likewise, an object denser than the fluid will sink. The buoyant force, which equals the weight of the fluid displaced, is thus greater than the weight of the object. This is because the fluid, having a higher density, contains more mass and hence more weight in the same volume. If its average density is less than that of the surrounding fluid, it will float. The average density of an object is what ultimately determines whether it floats. The buoyant force is always present whether the object floats, sinks, or is suspended in a fluid.ĭensity plays a crucial role in Archimedes’ principle. If the buoyant force equals the object’s weight, the object will remain suspended at that depth. If the buoyant force is less than the object’s weight, the object will sink. (See Figure 2.) If the buoyant force is greater than the object’s weight, the object will rise to the surface and float. There is a net upward, or buoyant force on any object in any fluid. This means that the upward force on the bottom of an object in a fluid is greater than the downward force on the top of the object. (credit: Crystl)Īnswers to all these questions, and many others, are based on the fact that pressure increases with depth in a fluid. (credit: Allied Navy) (c) Helium-filled balloons tug upward on their strings, demonstrating air’s buoyant effect. (b) Submarines have adjustable density (ballast tanks) so that they may float or sink as desired. (a) Even objects that sink, like this anchor, are partly supported by water when submerged. Note: You can enter whatever variables you know, and the calculator will compute the rest.Figure 1. The calculator will display the volume of the fluid displaced (2030 cm 3) and the buoyant force (19.89 N). If the height of the water inside the container changes by 2.86 cm, we can calculate the buoyant force as:Ĭlick on the Advanced mode button beneath the calculator and type the change in the height of the fluid (2.9 cm) and surface area of the fluid (700 cm 2).Įnter the true mass of the object (5 kg). Let us consider another example, where we drop a 5 kg rock into a cylindrical container with a base surface area of 700 cm 2. It will also show the buoyant force acting on the rock as 1.96 N and a message whether the rock will float or sink. The Archimedes' principle calculator will display the density of the rock as 2.70 g/cm 3 and the volume of the rock as 200 cm 3. You can also enter the density of the fluid manually. Using the drop-down menu, choose fluid type as water. We will calculate the average density of this rock as follows:Įnter the true mass and the apparent mass of the rock as 540 g and 340 g in the respective fields. Let the true mass (mass in the air) and apparent mass (when immersed in water) of a rock be 540 g and 340 g, respectively. In this section, we will try to determine the density of an unknown object using our Archimedes' principle calculator.


 0 kommentar(er)
0 kommentar(er)
